Maximum and minimum
[1][2][3] Pierre de Fermat was one of the first mathematicians to propose a general technique, adequality, for finding the maxima and minima of functions.A real-valued function f defined on a domain X has a global (or absolute) maximum point at x∗, if f(x∗) ≥ f(x) for all x in X.Similarly, the function has a global (or absolute) minimum point at x∗, if f(x∗) ≤ f(x) for all x in X.Symbolically, this can be written as follows: The definition of global minimum point also proceeds similarly.Similarly, the function has a local minimum point at x∗, if f(x∗) ≤ f(x) for all x in X within distance ε of x∗.In both the global and local cases, the concept of a strict extremum can be defined.An important example is a function whose domain is a closed and bounded interval of real numbers (see the graph above).Finding global maxima and minima is the goal of mathematical optimization.If a function is continuous on a closed interval, then by the extreme value theorem, global maxima and minima exist.So a method of finding a global maximum (or minimum) is to look at all the local maxima (or minima) in the interior, and also look at the maxima (or minima) of the points on the boundary, and take the greatest (or least) one.Minima For differentiable functions, Fermat's theorem states that local extrema in the interior of a domain must occur at critical points (or points where the derivative equals zero).feet of fencing and is trying to maximize the square footage of a rectangular enclosure, whereFor example, in the (enlargeable) figure on the right, the necessary conditions for a local maximum are similar to those of a function with only one variable.The first partial derivatives as to z (the variable to be maximized) are zero at the maximum (the glowing dot on top in the figure).These are only necessary, not sufficient, conditions for a local maximum, because of the possibility of a saddle point.For example, if a bounded differentiable function f defined on a closed interval in the real line has a single critical point, which is a local minimum, then it is also a global minimum (use the intermediate value theorem and Rolle's theorem to prove this by contradiction).This is illustrated by the function whose only critical point is at (0,0), which is a local minimum with f(0,0) = 0.The maximum and minimum function for sets are used in databases, and can be computed rapidly, since the maximum (or minimum) of a set can be computed from the maxima of a partition; formally, they are self-decomposable aggregation functions.Thus in a totally ordered set, we can simply use the terms minimum and maximum.




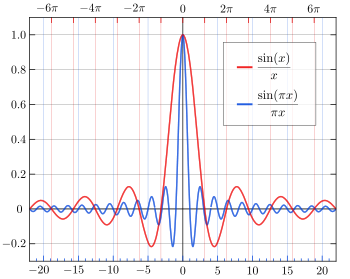
The unnormalised sinc function (red) has arg min of {−4.49, 4.49}, approximately, because it has 2 global minimum values of approximately −0.217 at x = ±4.49. However, the normalised sinc function (blue) has arg min of {−1.43, 1.43}, approximately, because their global minima occur at x = ±1.43, even though the minimum value is the same. [ 7 ]
Extreme value (disambiguation)Maximum (disambiguation)Minimum (disambiguation)mathematical analysisfunctiondomainPierre de Fermatadequalityset theorygreatest and least elementsinfinite setsreal numbersstatisticssample maximum and minimummetric spacetopological spacecontinuouscompactintervalmathematical optimizationextreme value theoremdifferentiable functionsFermat's theoremcritical pointsfirst derivative testhigher-order derivative testpiecewiseinflection pointsecond derivativestationary pointscritical pointendpointsSecond partial derivative testPeano surfacepartial derivativessaddle pointdifferentiableintermediate value theoremRolle's theoremcontradictionfunctionalcalculus of variationsordered setgreatest elementmaximal elementleast upper boundleast elementminimal elementgreatest lower bounddatabasesdecomposable aggregation functionspartial orderpartially ordered setupper boundtotally orderednatural numbersclosureArg maxmathematicsmaximized and minimizedargumentsdomain of a functioncodomainDerivative testInfimum and supremumLimit superior and limit inferiorMaximum-minimums identityMechanical equilibriumMex (mathematics)Stewart, JamesBrooks/ColeLarson, RonThomas, George B.Hass, JoelAddison-WesleyWayback Machinecritical valueEncyclopædia BritannicaCalculusPrecalculusBinomial theoremConcave functionContinuous functionFactorialFinite differenceFree variables and bound variablesGraph of a functionLinear functionRadianSecantTangentLimitsIndeterminate formLimit of a functionOne-sided limitLimit of a sequenceOrder of approximation(ε, δ)-definition of limitDifferential calculusDerivativePartial derivativeDifferentialDifferential operatorMean value theoremNotationLeibniz's notationNewton's notationRules of differentiationlinearityL'Hôpital'sProductGeneral Leibniz's ruleQuotientImplicit differentiationInverse functions and differentiationLogarithmic derivativeRelated ratesSecond derivative testNewton's methodTaylor's theoremDifferential equationOrdinary differential equationPartial differential equationStochastic differential equationIntegral calculusAntiderivative
