Anomaly (physics)
The most prevalent global anomaly in physics is associated with the violation of scale invariance by quantum corrections, quantified in renormalization.Global anomalies in symmetries that approach the identity sufficiently quickly at infinity do, however, pose problems.An exception may occur when the space of configurations is itself disconnected, in which case one may have the freedom to choose to integrate over any subset of the components.Thus when one sums over all physical configurations in the path integral, one finds that contributions come in pairs with opposite signs.The above description of a global anomaly is for the SU(2) gauge theory coupled to an odd number of (iso-)spin-1/2 Weyl fermion in 4 spacetime dimensions.[4] In 2018, it is found by Wang, Wen and Witten that the SU(2) gauge theory coupled to an odd number of (iso-)spin-3/2 Weyl fermion in 4 spacetime dimensions has a further subtler non-perturbative global anomaly detectable on certain non-spin manifolds without spin structure.[5] This new SU(2) anomaly also plays an important rule for confirming the consistency of SO(10) grand unified theory, with a Spin(10) gauge group and chiral fermions in the 16-dimensional spinor representations, defined on non-spin manifolds.It is found that the 4 dimensional pure Yang–Mills theory with only SU(2) gauge fields with a topological theta termQuantum anomalies were discovered via the process of renormalization, when some divergent integrals cannot be regularized in such a way that all the symmetries are preserved simultaneously.For example, the vanishing of the mixed anomaly involving two SU(2) generators and one U(1) hypercharge constrains all charges in a fermion generation to add up to zero,[10][11] and thereby dictates that the sum of the proton plus the sum of the electron vanish: the charges of quarks and leptons must be commensurate.There exists nonperturbative global anomalies classified by cyclic groups Z/nZ classes also known as the torsion part.It is widely known and checked in the late 20th century that the standard model and chiral gauge theories are free from perturbative local anomalies (captured by Feynman diagrams).There is also a formulation of both perturbative local and nonperturbative global description of anomaly inflow in terms of Atiyah, Patodi, and Singer [17] [18] eta invariant in one higher dimension.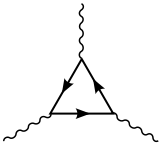
Quantum field theoryFeynman diagramHistoryField theoryElectromagnetismWeak forceStrong forceQuantum mechanicsSpecial relativityGeneral relativityGauge theoryYang–Mills theorySymmetriesSymmetry in quantum mechanicsC-symmetryP-symmetryT-symmetryLorentz symmetryPoincaré symmetryGauge symmetryExplicit symmetry breakingSpontaneous symmetry breakingNoether chargeTopological chargeBackground field methodBRST quantizationCorrelation functionCrossingEffective actionEffective field theoryExpectation valueLattice field theoryLSZ reduction formulaPartition functionPath Integral FormulationPropagatorQuantizationRegularizationRenormalizationVacuum stateWick's theoremWightman axiomsDirac equationKlein–Gordon equationProca equationsWheeler–DeWitt equationBargmann–Wigner equationsSchwinger-Dyson equationRenormalization group equationStandard ModelQuantum electrodynamicsElectroweak interactionQuantum chromodynamicsHiggs mechanismString theorySupersymmetryTechnicolorTheory of everythingQuantum gravityAndersonAnselmBargmannBecchiBelavinBerezinBjorkenBleuerBogoliubovBrodskyBuchholzCachazoCallanColemanConnesDashenDeWittDoplicherEnglertFaddeevFeynmanFramptonFritzschFröhlichFredenhagenGlashowGell-MannGoldstoneGribovGuralnikHeisenberg't HooftIliopoulosIvanenkoJackiwJona-LasinioJordanKällénKendallKinoshitaKlebanovKontsevichKreimerKuraevLandauLehmannLeutwylerLipatovŁopuszańskiLüdersMaianiMajoranaMaldacenaMatsubaraMigdalMøllerNaimarkNishijimaOppenheimerOsbornOsterwalderParisiPecceiPeskinPlefkaPolchinskiPolyakov