Klein bottle
Note that this is an "abstract" gluing in the sense that trying to realize this in three dimensions results in a self-intersecting Klein bottle.[2] To construct the Klein bottle, glue the red arrows of the square together (left and right sides), resulting in a cylinder.For example, the Klein bottle has no boundary, where the surface stops abruptly, and it is non-orientable, as reflected in the one-sidedness of the immersion.The Science Museum in London has a collection of hand-blown glass Klein bottles on display, exhibiting many variations on this topological theme.Gently push a piece of the tube containing the intersection along the fourth dimension, out of the original three-dimensional space.After the figure has grown for a while, the earliest section of the wall begins to recede, disappearing like the Cheshire Cat but leaving its ever-expanding smile behind.[4] Continuing this sequence, for example creating a 3-manifold which cannot be embedded in R4 but can be in R5, is possible; in this case, connecting two ends of a spherinder to each other in the same manner as the two ends of a cylinder for a Klein bottle, creates a figure, referred to as a "spherinder Klein bottle", that cannot fully be embedded in R4.The Klein bottle can be constructed (in a four dimensional space, because in three dimensional space it cannot be done without allowing the surface to intersect itself) by joining the edges of two Möbius strips, as described in the following limerick by Leo Moser:[6] A mathematician named Klein Thought the Möbius band was divine.The parameter θ gives the angle in the xy plane as well as the rotation of the figure 8, and v specifies the position around the 8-shaped cross section.A non-intersecting 4-D parametrization can be modeled after that of the flat torus: where R and P are constants that determine aspect ratio, θ and v are similar to as defined above.The v bump causes the self intersecting 2-D/planar figure-8 to spread out into a 3-D stylized "potato chip" or saddle shape in the x-y-w and x-y-z space viewed edge on.If the traditional Klein bottle is cut in its plane of symmetry it breaks into two Möbius strips of opposite chirality.A figure-8 Klein bottle can be cut into two Möbius strips of the same chirality, and cannot be regularly deformed into its mirror image.[10] In another order of ideas, constructing 3-manifolds, it is known that a solid Klein bottle is homeomorphic to the Cartesian product of a Möbius strip and a closed interval.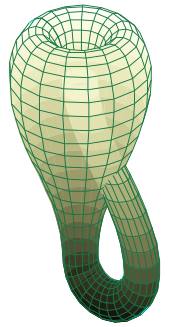









immersedmathematicsnon-orientablesurfacetwo-dimensionalmanifoldnormal vectorcontinuouslyMöbius stripreal projective planeboundarysphereFelix Kleinfundamental polygonimmersionthree-dimensional spaceScience Museum in LondonTime evolutionCheshire Catquotient spacesquareorientablecompactEuclidean spacespherinderfiber bundlecirclelimerickLeo MoserCW complexEuler characteristichomology groupscovering mapfundamental regionuniversal coverfundamental grouppresentationHeawood conjecturefour color theoremconnected sumprojective planescross-capsplane of symmetryMöbius stripslemniscate of GeronoLissajous curvepinch pointsregular homotopyachiral3-manifoldssolid Klein bottlehomeomorphicCartesian productRiemann surfacestransition mapscomplex conjugationdianalytic structureAlgebraic topologyAlice universeBoy's surfaceMarc ten BoschCiteSeerXPlanetMathWeisstein, Eric W.MathWorldBulletin of the American Mathematical SocietyStillwell, JohnSpringer-VerlagXScreenSaverRoman surfaceDyck's surfaceAnnulusCylinderCross-capSphere with three holesConnectednessCompactnessTriangulatednesssmoothnessOrientabilityhandleManifoldsGlossaryTopological manifoldDifferentiable/Smooth manifoldDifferential structureSmooth atlasSubmanifoldRiemannian manifoldSmooth mapSubmersionPushforwardTangent spaceDifferential formVector fieldAtiyah–Singer indexDarboux'sFrobeniusGeneralized StokesHopf–RinowNoether'sSard'sWhitney embeddingDiffeomorphismGeodesicExponential mapin Lie theoryFoliationIntegral curveLie derivativeSectionClosedCollapsingAlmostComplexContactFiberedFinslerG-structureHadamardHermitianHyperbolicKählerKenmotsuLie groupLie algebra